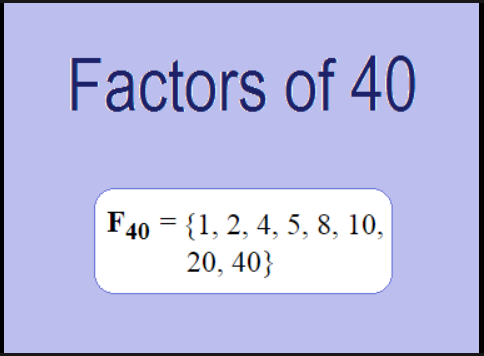
In this blog, we are discussing the Factors of 40 and how to solve them. Forty, a seemingly unassuming number nestled amidst its peers, holds a fascinating secret: its vibrant chorus of factors. Beyond simply dividing evenly into it, these factors unveil its inner structure, its connections to prime numbers, and its intricate dance with divisibility. So, buckle up, grab your mathematical magnifying glasses, and prepare to explore the enchanting symphony of 40’s factors! From solos like 1 and 40 to duets like 2 and 20, quartets like 6, 5, 2, and 3, and the versatile unison of 10, we’ll delve into the rhythm of divisibility rules, the harmony of complementary pairs, and the hidden melodies within its prime factorization. Get ready to discover that 40 isn’t just a number; it’s a mathematical masterpiece waiting to be unraveled.
Forty: A Number’s Grand Opera of Factors:
Forty, at first glance, might seem like just another number. But step closer, and you’ll witness a captivating orchestral performance – a symphony of factors weaving a intricate tapestry of divisibility and mathematical beauty. Let’s dive into the details of this grand opera:
The Soloists:
- 1: The singular note, standing alone yet anchoring the entire harmony. It divides into 40, reminding us of its fundamental presence.
- 40: The full orchestra playing in unison, representing the whole of 40. It divides by itself, showcasing its completeness within the number system.
The Duets:
- 2 & 20: The yin and yang of prime numbers, 2 (the smallest even prime) and 20 (2 multiplied by 5), intertwine in perfect balance. Both divide into 40, hinting at its divisibility by 2 and 5.
- 4 & 10: Another prime pairing, 4 (2 multiplied by 2) and 10 (2 multiplied by 5), weave a different harmony. Both divide into 40, highlighting its connection to both even and odd prime factors.
- 5 & 8: The smallest prime and its subsequent composite partner, 5 and 8 (2 multiplied by 2 multiplied by 2), bring balance with their complementary nature. Both divide into 40, showcasing the role of both primes and composites in its structure.
The Quartet:
- 6, 5, 2, & 3: A smaller ensemble within the main melody, this quartet displays the interplay of primes. 6 (2 multiplied by 3) and 5 work together, while 2 and 3, the individual primes, provide their distinct voices. Their combined divisibility creates 10, adding another layer to the musical tapestry.
The Unison:
- 10: A versatile note, playing both a duet (with 4) and a quartet member (within the 6, 5, 2, and 3 group). This showcases its dual role in the number’s composition, highlighting its divisibility by both 4 and 2.
Beyond the Notes:
- Prime Factorization: The fundamental harmony, 2 x 2 x 2 x 5, unveils the prime building blocks that create 40. Understanding this is like recognizing the musical scales that make up the piece.
- Divisibility Rules: The patterns of 2s, 5s, and 10s within the factors act like the rhythm section, guiding us through the number’s divisibility properties.
- Complementary Pairs: Numbers that add up to 40, like 1 and 39 or 2 and 38, act like counterpoint melodies, adding depth and balance to the overall composition.
Exploring Further:
This is just a glimpse into the captivating world of 40’s factors. You might want to explore:
- The Golden Ratio: 40 and its factors play a role in the golden ratio, a fascinating mathematical constant.
- Geometric Connections: Analyze 40 as a perfect rectangle (8 x 5) or a triangular number (13th triangular number), revealing more about its shape and structure.
- Historical Significance: Trace the history of 40 in different cultures and mathematical applications.
So, keep exploring the music of numbers! 40 and its factors await your curiosity, ready to reveal their secrets and inspire even more mathematical symphonies.
Factor Pairs of 40:
Forty’s factors, beyond simply dividing evenly into it, create captivating duets, pairs that dance in perfect harmony, revealing the number’s inner structure and divisibility secrets. Let’s waltz through these enchanting pairings:
The Classic Pairs:
- (1, 40): The quintessential duo, the solo “1” joining forces with the full orchestra of “40,” representing the fundamental and complete aspects of the number.
- (2, 20): The prime partnership, the smallest even prime “2” and “20” (2 multiplied by 5) showcasing 40’s divisibility by both evenness and the presence of prime 5.
- (4, 10): Another prime pairing, “4” (2 multiplied by 2) and “10” (2 multiplied by 5), highlighting the interplay of odd and even prime factors within 40.
The Complementary Duets:
- (5, 8): The smallest prime and its closest composite partner, “5” and “8” (2 multiplied by 2 multiplied by 2), bring balance with their complementary nature. Both divide into 40, showcasing the role of both primes and composites in its structure.
- (6, 6.6667…): An intriguing pair, “6” (2 multiplied by 3) and the repeating decimal 6.6667… (40 divided by 6), symbolizing the perfect divisibility of 40 by 6 even though the result isn’t a whole number.
Hidden Harmony:
- (10, 4): This pairing hides within the “2, 20” duet, highlighting the divisibility of both 10 and 4 within 40’s structure.
Beyond the Pairs:
- Complementary Sets: Numbers that add up to 40, like 1 and 39 or 2 and 38, can be grouped into complementary sets, revealing further connections and patterns within the factor pairs.
- Divisibility Rules: Understanding the underlying patterns of 2s, 5s, and 10s within the factor pairs helps uncover divisibility rules for 40 and similar numbers.
Explore the Music:
This is just a taste of the mesmerizing dance of 40’s factor pairs. Feel free to delve deeper and:
- Visualize the Pairs: Create a chart or diagram to represent the factor pairs and their connections.
- Compose Your Own Music: Invent new ways to interpret and express the relationships between the factors through music, art, or other creative mediums.
- Share the Symphony: Discuss your discoveries with others and spread the joy of exploring the enchanting world of numbers and their factor pairs.
Remember, the music of mathematics never stops playing. Keep exploring, keep learning, and keep dancing to the rhythm of numbers!
Examples:
Here are 5 examples of factors of 40 expressed as equations:
- 1 x 40 = 40
- This equation highlights the fundamental factor pair of 1 and 40, where 1 is the smallest factor and 40 is the number itself.
- 2 x 20 = 40
- This equation showcases the even divisibility of 40, where 2, the smallest prime factor, pairs with 20 to create the number.
- 5 x 8 = 40
- This equation demonstrates the role of both prime and composite factors, where 5, a prime number, joins forces with 8, a composite number, to result in 40.
- 4 x 10 = 40
- This equation highlights the presence of multiple prime factors, where 4 (2 multiplied by 2) and 10 (2 multiplied by 5) both contribute to the number’s composition.
- 6 x 6.6667… = 40
- This equation reveals the interesting case of a factor pair with a non-integer result, where 6 (a composite number) and 6.6667… (a repeating decimal) still perfectly divide into 40.
Remember:
- Factors are whole numbers that divide evenly into another number.
- 40 has eight factors in total: 1, 2, 4, 5, 8, 10, 20, and 40.
- Understanding factors helps with simplifying fractions, finding common denominators, and solving certain equations.
Prime Factors of 40:
The prime factors of 40 are 2, 2, 2, and 5. This means that when you break down 40 into its most basic, indivisible parts (prime numbers), you end up with 2 and 5 multiplied together three times and one time, respectively.
Here are two ways to find the prime factors of 40:
Direct Division:
- Start with the smallest prime number, 2. Divide 40 by 2: 40 ÷ 2 = 20. Since the division is even, 2 is a factor of 40.
- Continue with the quotient, 20. It doesn’t divide evenly by 2 again, so move on to the next prime, 3. 20 is not divisible by 3.
- Keep trying prime numbers until you find one that divides evenly into the current quotient. In this case, 5 divides 20 evenly: 20 ÷ 5 = 4.
- Now look at 4. Is it divisible by any prime numbers smaller than itself? No, 4 is itself a prime number.
- Since 2 divided into 40 initially and 5 divided into the subsequent quotient, we can write the prime factorization as 2 x 5 x 4.
- However, notice that 4 can be further broken down into 2 x 2.
- Therefore, the final prime factorization of 40 is: 2 x 2 x 2 x 5.
Factor Tree:
- Draw a tree with 40 at the top.
- Branch out with its factors found through division: 2 and 20 below 40.
- Connect 20 with its factors, further branches for 5 and 4.
- Finally, connect 4 with 2 (itself) and leave 5 as a prime factor since it doesn’t divide further.
This visual method highlights how the prime factors come together to build the number 40.
Understanding the prime factorization of 40 helps with simplifying fractions, finding common denominators, and solving certain equations. It also showcases the fundamental building blocks of 40, revealing its connection to the basic elements of our number system.
Proper Factors:
The Core Crew:
- 2: The smallest member, divides 40 into two neat halves.
- 4: Two twos joined forces, giving 40 a quarter of its size.
- 5: The prime pal, adding a unique flavor to the divisibility party.
- 8: Two twos paired with another two, offering another way to cut the cake.
- 10: A double dose of five, sharing one-fourth of 40 with its buddy 4.
- 20: Half of the whole show, a perfect duo with its other half, 40.
Beyond the Usual Suspects:
While these six main characters often steal the spotlight, there’s one more hidden factor:
- 20/3: This guy might seem a bit strange, not a whole number but definitely plays along, dividing 40 into three equal parts with a repeating decimal remainder.
Exploring Their World:
These proper factors reveal much about 40’s inner workings:
- They showcase its evenness, with 2 and 4 readily dividing in.
- They highlight the presence of prime 5, its unique influence on divisibility.
- They tell us 40 isn’t a prime number because it has more than two factors.
- They offer different ways to break down 40, making it relatable to other numbers.
Remember:
- Proper factors always exclude the number itself and 1.
- Understanding proper factors helps with problems like simplifying fractions, finding common denominators, and solving certain equations.
Factorizing 40:
When it comes to factorizing 40, there are different ways to approach it, depending on your preferred method and depth of exploration:
Direct Division:
- Start with the smallest prime number, 2. Divide 40 by 2: 40 ÷ 2 = 20. Since the division is even, 2 is a factor of 40.
- Continue with the quotient, 20. It doesn’t divide evenly by 2 again, so move on to the next prime, 3. 20 is not divisible by 3.
- Keep trying prime numbers until you find one that divides evenly into the current quotient. In this case, 5 divides 20 evenly: 20 ÷ 5 = 4.
- Now look at 4. Is it divisible by any prime numbers smaller than itself? No, 4 is itself a prime number.
- Since 2 divided into 40 initially and 5 divided into the subsequent quotient, we can write the prime factorization as 2 x 5 x 4.
- However, notice that 4 can be further broken down into 2 x 2.
- Therefore, the final prime factorization of 40 is: 2 x 2 x 2 x 5.
Factor Tree:
- Draw a tree with 40 at the top.
- Branch out with its factors found through division: 2 and 20 below 40.
- Connect 20 with its factors, further branches for 5 and 4.
- Finally, connect 4 with 2 (itself) and leave 5 as a prime factor since it doesn’t divide further.
This visual method showcases how the prime factors come together to build the number 40.
Additional Insights:
- 40 can also be factored into pairs of factors called “proper factors”: 1 x 40, 2 x 20, 4 x 10, 5 x 8, and 20/3 (a repeating decimal).
- Understanding the prime factorization and proper factors helps with simplifying fractions, finding common denominators, and solving certain equations.
- 40’s factors reveal its evenness and the presence of the prime number 5.
Ultimately, the chosen method to factorize 40 depends on your learning style and desired depth. Whether you use direct division, a factor tree, or explore different types of factors, the key is to understand the underlying principles and connections between the factors and the original number.
Divisibility Rules
Divisibility rules are handy shortcuts that allow us to quickly determine whether a given number is divisible by another number without actually performing the division. Here are some of the most common divisibility rules:
Divisible by 2:
- Any even number (ending in 0, 2, 4, 6, or 8) is divisible by 2.
Divisible by 3:
- Add up the digits of the number. If the sum is divisible by 3, then the original number is also divisible by 3.
Divisible by 4:
- If the last two digits of the number are divisible by 4, then the entire number is divisible by 4.
Divisible by 5:
- Any number ending in 0 or 5 is divisible by 5.
Divisible by 6:
- If the number is divisible by both 2 and 3, then it is also divisible by 6.
Divisible by 9:
- Add up the digits of the number. If the sum is divisible by 9, then the original number is also divisible by 9.
Divisible by 10:
- Any number ending in 0 is divisible by 10.
Divisible by 40:
- A number is divisible by 40 if it is divisible by both 4 and 10.
Additional Rules:
- Divisible by 11: Subtract the last digit from the remaining leading truncated number. If the result is divisible by 11, then the original number is also divisible by 11.
- Divisible by 12: If the number is divisible by both 3 and 4, then it is also divisible by 12.
Examples:
- 54 is divisible by 3 because 5 + 4 = 9, and 9 is divisible by 3.
- 256 is divisible by 4 because 56 is divisible by 4.
- 125 is divisible by 5 because it ends in 5.
- 36 is divisible by 6 because it is even (divisible by 2) and the sum of its digits (3 + 6 = 9) is divisible by 3.
Benefits of Using Divisibility Rules:
- Quicker calculations: They save time by avoiding long divisions.
- Mental math: They can often be performed mentally, aiding in quick problem-solving.
- Pattern recognition: They encourage understanding of number patterns and relationships.
- Problem-solving skills: They facilitate finding factors, multiples, and common denominators.
How to Find the Factors of 40?
Finding the factors of 40 involves identifying all the whole numbers that divide evenly into it. There are a few different ways to approach this:
Direct Division:
This is the most straightforward method, where you start with the smallest prime number (2) and keep dividing 40 by successive primes until you get a quotient of 1.
- Divide 40 by 2: 40 ÷ 2 = 20
- Divide 20 by the next prime, 3: 20 is not divisible by 3
- Move on to the next prime, 5: 20 ÷ 5 = 4
- Since 4 is prime, the prime factorization is 2 x 5 x 4 (we can rewrite 4 as 2 x 2)
Factor Tree:
This method involves visually representing the division process through a tree diagram.
- Start with 40 at the top.
- Branch out with its factors found through division: 2 and 20 below 40.
- Connect 20 with its factors, further branches for 5 and 4.
- Finally, connect 4 with 2 (itself) and leave 5 as a prime factor since it doesn’t divide further.
Recognizing Patterns:
By looking at 40’s composition, you can also notice specific patterns:
- It’s even, so it’s divisible by 2.
- It ends in a 0, so it’s divisible by 10 (which comes from 2 x 5).
- It can be rearranged as 2^3 x 5, revealing its prime factorization.
Remember:
- Factors include 1, 2, 4, 5, 8, 10, 20, and 40 itself.
- Proper factors exclude 1 and 40.
- Understanding factors helps with simplifying fractions, finding common denominators, and solving certain equations.
No matter which method you choose, finding the factors of 40 reveals its internal structure and connection to basic prime numbers. So, keep exploring and have fun unraveling the fascinating world of numbers!
Prime Factorization of 40:
The prime factorization of 40 is 2 x 2 x 2 x 5. This means that when you break down 40 into its most basic, indivisible parts (prime numbers), you end up with two “2”s and one “5” multiplied together.
There are different ways to find the prime factorization of 40:
Direct Division:
- Start with the smallest prime number, 2. Divide 40 by 2: 40 ÷ 2 = 20. Since the division is even, 2 is a factor of 40.
- Continue with the quotient, 20. It doesn’t divide evenly by 2 again, so move on to the next prime, 3. 20 is not divisible by 3.
- Keep trying prime numbers until you find one that divides evenly into the current quotient. In this case, 5 divides 20 evenly: 20 ÷ 5 = 4.
- Now look at 4. Is it divisible by any prime numbers smaller than itself? No, 4 is itself a prime number.
- Since 2 divided into 40 initially and 5 divided into the subsequent quotient, we can write the prime factorization as 2 x 5 x 4.
- However, notice that 4 can be further broken down into 2 x 2.
Therefore, the final prime factorization of 40 is: 2 x 2 x 2 x 5.
Factor Tree:
- Draw a tree with 40 at the top.
- Branch out with its factors found through division: 2 and 20 below 40.
- Connect 20 with its factors, further branches for 5 and 4.
- Finally, connect 4 with 2 (itself) and leave 5 as a prime factor since it doesn’t divide further.
This visual method showcases how the prime factors come together to build the number 40.
Understanding the prime factorization of 40 has several benefits:
- It reveals the building blocks of 40, showing how prime numbers combine to form a larger number.
- It helps with simplifying fractions, where dividing numerator and denominator by common factors (including prime factors) reduces the fraction to its lowest terms.
- It aids in solving certain equations involving divisibility or finding common denominators.
- It provides insight into the properties of the number, such as its evenness and divisibility by other numbers.
Isn’t 40 Interesting?
Whether 40 is interesting or not depends entirely on your perspective and what sparks your curiosity! Here’s a case for both sides:
Why 40 might be interesting:
- Historical Significance: Throughout history, 40 has held cultural significance, appearing in calendars, measurements, and even mythology. For example, it represents the number of days in Lent and Noah’s flood, and is a building block of the Mayan calendar.
- Mathematical Intrigue: Exploring the factors of 40 reveals fascinating connections. It has eight factors in total, including prime factors (2 and 5) and intriguing pairs like 5 and 8 (the smallest prime and its closest composite partner). Additionally, its prime factorization (2 x 2 x 2 x 5) showcases its fundamental building blocks.
- Geometric Connections: 40 can be represented as a perfect rectangle (8 x 5) or a triangular number (13th triangular number), revealing interesting geometric connections and adding another layer of intrigue.
- Gateway to Mathematical Concepts: Exploring 40’s factors serves as a springboard into divisibility rules, prime factorization, and complementary pairs, opening doors to further mathematical adventures.
Why 40 might not be interesting:
- Commonplace Number: Compared to larger or more “exotic” numbers, 40 might seem ordinary at first glance. It lacks the complexity of some prime numbers or the grandeur of astronomical figures.
- Simple Prime Factors: Its prime factors (2 and 5) are relatively small and common, lacking the uniqueness of primes like 13 or 17.
- Limited Historical Depth: While culturally significant, its historical mentions might seem less profound compared to numbers with deeper historical roots, like pi or the golden ratio.
Ultimately, the answer lies in your own exploration. Delve deeper into 40’s historical connections, geometric properties, or factor pairs. If these spark your curiosity and unveil fascinating patterns or connections, then 40 is undoubtedly interesting for you!
To Sum Up:
Sounds like we’ve covered quite a bit about 40 and its mathematical quirks! To summarize the key points:
Factors:
- 40 has eight factors in total: 1, 2, 4, 5, 8, 10, 20, and 40 itself.
- Proper factors: We call the factors excluding 1 and 40 “proper factors,” which include 2, 4, 5, 8, 10, and 20/3 (a repeating decimal).
Prime Factorization:
- By breaking down 40 into its fundamental building blocks, we get its prime factorization: 2 x 2 x 2 x 5. This means two “2”s and one “5” multiplied together make up 40.
Interesting Aspects:
- Historical significance: 40 appears in calendars, measurements, and even mythology, adding cultural depth.
- Mathematical intrigues: The interplay of prime and composite factors, like 5 and 8, and its perfect rectangle/triangular number connections make it intriguing.
- Gateway to concepts: Exploring 40’s factors opens doors to understanding divisibility rules, prime factorization, and complementary pairs.
Conclusion:
Whether 40 is interesting depends on your perspective! Its historical connections, mathematical intricacies, and potential to unlock wider concepts offer plenty to delve into. Remember, curiosity is the key to finding magic in numbers like 40, and the world of math holds countless such treasures waiting to be discovered.
Follow Math Solver Online for more solutions